P^2+Σ2P

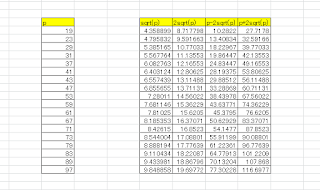
Elliptic curves have an endomorphism ring larger than the integers, which are higher dimensions of abelian varieties. C/ΘZ[i] C is complex multiplication, and Z[i] is the Gaussian integer ring, and θ is any non-zero complex number. You can see always the Gaussian integer as module in Elliptic curves. Y^2=X^3-X+1 59 is the Gaussian prime. The Gaussian prime must be symmetry in any Elliptic curves. 2=(1-i)(1+i)=(-1-i)(-1+i) This is the norm. 1±i,-1±i are Gaussian primes. ±3,±3i,5=(2-i)(2+i)=(1-2i)(1+2i),±7,±7i,13=(2+3i)(2-3i)=(3+2i)(3-2i),17=(4-i)(4+i)=(1+4i)(1-4i) Y^2=4X^3-X 59 is the Gaussian prime. I think that it is fractal .

This is quite tricky. Modulo primes in Y^2=X^3-X are sometimes primes . p-2sqrt(p) p is prime, and sqrt is √ When p is 19, N is 19. p=23 - N=23 p=29 - N=39 Carl Friedrich Gauss proved it. Moreover, p=N is Gaussian primes such as 19 and 23. This is Gaussian integers which are complex numbers. Elliptic curve is rational, so this is contradictory but prime is real. 5=(2+i)(2-i) You can divide 5 in Z[i], so 5 isn't prime. However, 5 is prime in the real world. This is Fermat's theorem on sums of two squares. p≡1 (mod 4) ex. 5,13=(2+3i)(2-3i),17=(1+4i)(1-4i),29=(2+5i)(2-5i) When 3=αβ, this isn't also prime in Z[i]. N is the norm. N(a+bi):=a^2+b^2=(a+bi)(a-bi) N(αβ)=N(α)N(β) 3≠(a+bi)(a-bi) Therefore, 3 is prime in Z[i]. 4n+3 is Gaussian primes such as 3,7,11,19,23,31,43,47,59,67,71,79,83. This is in Y^2=X^3-X. E(N)=G(p)≒4n+3≠(a+bi)(a-bi) E(N) is the sum of the same modules in Y^2=X^3-X. G(p) is Gaussian primes. You see COUNTIF(mod(y^2),mod(...