This is the same process with Y^2=X^3-X but the different elliptic curve. This is still fractal. n=5 is the first congruent number. n is the integer without square factors.
You can also write this. n is Congruent number.
ab/2=n
(a/c)^2+(b/c)^2=1
This is rational.
a=cx, b=cy
You remember rational point in X^2+y^2=1. This is expansion, but you select proper numbers. (n=5,6,7,13,14,15,20,95)
You see the horizon which is 0. Y^2=X^3-n^2X
(n=157)











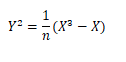


0 件のコメント:
コメントを投稿